Fractals Are Everywhere

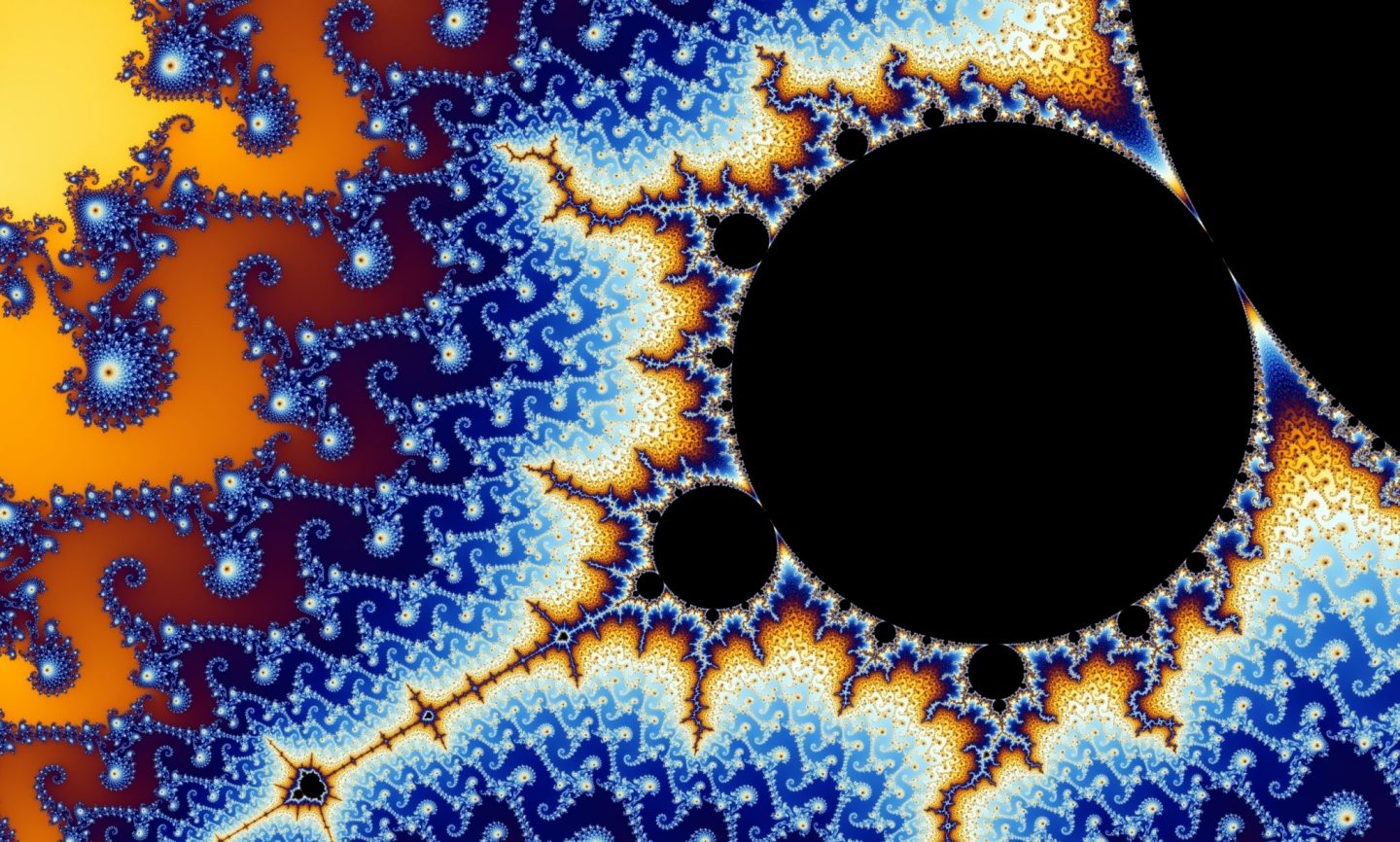
Fractal comes from the Latin word fractus (translated as ‘fractured’) and it refers to any irregular, ‘fractured’ looking shape. The term was coined by Benoit Mandelbrot, the IBM mathematician who first produced computer-generated images of fractals and mathematically interpreted nature in his book The Fractal Geometry of Nature. The most famous computer-generated fractal shape is the Mandelbrot set, related to the Julia set, and its infinite detail and depth can be described by a very simple equation. A value is fed into the equation and the result is then fed back into the equation – it is this recursive nature of the equation which leads to the infinite nature of the fractal image.

                                 Mandelbrot set

It is interesting that fractals have existed in nature, yet no-one paid any real, scientific attention to them until the 1980s. This goes to show that scientific and mathematical breakthroughs heavily depend on circumstance. Fractals in nature could only really be understood, mathematically, once computers were powerful enough to generate fractal images. This is not to say though that an essential feature of fractals, self-similarity, had not been understood in the past. Self-similarity is when the parts of a shape resemble the whole shape or are a copy of the whole shape. The Cantor Set and the Koch snowflake are prime examples of this.

               Koch snowflake, full of repeating triangles

In fact, some artists have even used self-similarity in their art; for example, the Japanese artist Hokusai used the repeating pattern of waves in his well-known painting The Great Wave Off Kanagawa.

A lot of religious art, particularly art from Eastern religions, make use of self-similarity and bear some similarities to fractals. Tibetan thangka paintings would be a good example of this.

Fractal geometry seems to act as a blueprint for many living things. In nature, fractals are everywhere. The centre of sunflowers, pine cones, ferns, the shape of lightning and river meanderings, the branching of trees, veins and blood vessels, lungs, and the countless other forms of other countless plants and animals. It has been said that nature has exploited fractal geometry because it is the most efficient way for something to grow or because it is the easiest way to increase surface area (making it easier for the lungs to absorb oxygen, for example).

                                          The lungs of a dog

My favourite example of a fractal in nature is the one that most resembles a computer-generated fractal, called the Romanesco broccoli. It is easy to see the repeating pattern of cones. As a whole, the cauliflower is a large cone, but at any point is another cone, which contains cones within cones etc. It’s dizzying. But if you want to appreciate how infinite complexity can arise from simple rules, watch videos of ‘fractal zooming’ on YouTube.

                                      Romanesco broccoli

The study of fractals in the 80s and the later computer-generated images of the Mandelbrot set spawned a whole new type of art called fractal art.

                                                  Fractal art

It’s interesting that a kind of mathematics, belonging to a kind of science (the science of chaos) would lead to a new aesthetic appreciation of fractals, shapes which have always existed in nature. Perhaps the psychedelic art of the 60s though did pre-empt this in some way, since tie-dye and kaleidoscopic art is very similar to fractal art. It is also supposed to be very common to see images of fractals under the influence of LSD, psilocybin, mescaline and DMT.

                                                       DMT-inspired art

This seems pretty strange. How could a drug make you see an image of a fractal? Does the drug make your visual system recursive, so that shapes and colours are fed into themselves, eventually producing a fractal image? If that’s what happens, how and why does the drug do this? High doses of these drugs can make users feel connected with nature, so maybe seeing fractals is part of the visual aspect of that experience, since fractals do have their basis in nature itself.

Fractal geometry now has a wide-range of applications. They are being applied to computer chips so that they can store more information and in computer-generated landscapes for films such as Star Wars and Star Trek. It was only because of fractal geometry that computers were able to generate images of mountains and forests which had that realistic, fractured, irregular shape. There are many other useful applications in video game design, engineering, medicine and other areas of technology. Fractal geometry is a very recent kind of geometry so its implications for the future are not clear.

                       Computer-generated fractal landscape

It may even be relevant to physics and our view of reality. Arthur C. Clarke (author of 2001: A Space Odyssey), in a discussion with Carl Sagan and Stephen Hawking (which is on YouTube) asked Stephen Hawking if the fundamental nature of reality could be fractal. In other words, if we keep zooming deeper and deeper into matter, will we reach some smallest, fundamental particle or string (if string theory becomes verified in the future) or will the zooming go on forever? Hawking dismissed the idea by saying that the deepest level of matter is the Planck length – nothing can be smaller than this length.

But maybe as particle colliders become more sophisticated, it will become apparent that material objects are like fractals, in that they are finite in extent, but they are infinite in depth. In other words, the object takes up a limited amount of space, but the depth of the object is never-ending. You could zoom into it forever. Even if this does not turn out to be true, it’s still a fascinating fact that fractals are both finite and infinite in this way.